Essays on Education and Kindred Subjects

On further considering the matter, however, it will perhaps be felt that this definition does not express the whole fact – that inseparable as science may be from common knowledge, and completely as we may fill up the gap between the simplest previsions of the child and the most recondite ones of the natural philosopher, by interposing a series of previsions in which the complexity of reasoning involved is greater and greater, there is yet a difference between the two beyond that which is here described. And this is true. But the difference is still not such as enables us to draw the assumed line of demarcation. It is a difference not between common knowledge and scientific knowledge; but between the successive phases of science itself, or knowledge itself – whichever we choose to call it. In its earlier phases science attains only to certainty of foreknowledge; in its later phases it further attains to completeness. We begin by discovering a relation: we end by discovering the relation. Our first achievement is to foretell the kind of phenomenon which will occur under specific conditions: our last achievement is to foretell not only the kind but the amount. Or, to reduce the proposition to its most definite form – undeveloped science is qualitative prevision: developed science is quantitative prevision.

This will at once be perceived to express the remaining distinction between the lower and the higher stages of positive knowledge. The prediction that a piece of lead will take more force to lift it than a piece of wood of equal size, exhibits certainty, but not completeness, of foresight. The kind of effect in which the one body will exceed the other is foreseen; but not the amount by which it will exceed. There is qualitative prevision only. On the other hand, the prediction that at a stated time two particular planets will be in conjunction; that by means of a lever having arms in a given ratio, a known force will raise just so many pounds; that to decompose a specified quantity of sulphate of iron by carbonate of soda will require so many grains – these predictions exhibit foreknowledge, not only of the nature of the effects to be produced, but of the magnitude, either of the effects themselves, of the agencies producing them, or of the distance in time or space at which they will be produced. There is not only qualitative but quantitative prevision.

And this is the unexpressed difference which leads us to consider certain orders of knowledge as especially scientific when contrasted with knowledge in general. Are the phenomena measurable? is the test which we unconsciously employ. Space is measurable: hence Geometry. Force and space are measureable: hence Statics. Time, force, and space are measureable: hence Dynamics. The invention of the barometer enabled men to extend the principles of mechanics to the atmosphere; and Aerostatics existed. When a thermometer was devised there arose a science of heat, which was before impossible. Such of our sensations as we have not yet found modes of measuring do not originate sciences. We have no science of smells; nor have we one of tastes. We have a science of the relations of sounds differing in pitch, because we have discovered a way to measure them; but we have no science of sounds in respect to their loudness or their timbre, because we have got no measures of loudness and timbre.

Obviously it is this reduction of the sensible phenomena it represents, to relations of magnitude, which gives to any division of knowledge its especially scientific character. Originally men's knowledge of weights and forces was in the same condition as their knowledge of smells and tastes is now – a knowledge not extending beyond that given by the unaided sensations; and it remained so until weighing instruments and dynamometers were invented. Before there were hour-glasses and clepsydras, most phenomena could be estimated as to their durations and intervals, with no greater precision than degrees of hardness can be estimated by the fingers. Until a thermometric scale was contrived, men's judgments respecting relative amounts of heat stood on the same footing with their present judgments respecting relative amounts of sound. And as in these initial stages, with no aids to observation, only the roughest comparisons of cases could be made, and only the most marked differences perceived; it is obvious that only the most simple laws of dependence could be ascertained – only those laws which, being uncomplicated with others, and not disturbed in their manifestations, required no niceties of observation to disentangle them. Whence it appears not only that in proportion as knowledge becomes quantitative do its previsions become complete as well as certain, but that until its assumption of a quantitative character it is necessarily confined to the most elementary relations.

Moreover it is to be remarked that while, on the one hand, we can discover the laws of the greater proportion of phenomena only by investigating them quantitatively; on the other hand we can extend the range of our quantitative previsions only as fast as we detect the laws of the results we predict. For clearly the ability to specify the magnitude of a result inaccessible to direct measurement, implies knowledge of its mode of dependence on something which can be measured – implies that we know the particular fact dealt with to be an instance of some more general fact. Thus the extent to which our quantitative previsions have been carried in any direction, indicates the depth to which our knowledge reaches in that direction. And here, as another aspect of the same fact, we may further observe that as we pass from qualitative to quantitative prevision, we pass from inductive science to deductive science. Science while purely inductive is purely qualitative: when inaccurately quantitative it usually consists of part induction, part deduction: and it becomes accurately quantitative only when wholly deductive. We do not mean that the deductive and the quantitative are coextensive; for there is manifestly much deduction that is qualitative only. We mean that all quantitative prevision is reached deductively; and that induction can achieve only qualitative prevision.

Still, however, it must not be supposed that these distinctions enable us to separate ordinary knowledge from science, much as they seem to do so. While they show in what consists the broad contrast between the extreme forms of the two, they yet lead us to recognise their essential identity; and once more prove the difference to be one of degree only. For, on the one hand, the commonest positive knowledge is to some extent quantitative; seeing that the amount of the foreseen result is known within certain wide limits. And, on the other hand, the highest quantitative prevision does not reach the exact truth, but only a very near approximation to it. Without clocks the savage knows that the day is longer in the summer than in the winter; without scales he knows that stone is heavier than flesh: that is, he can foresee respecting certain results that their amounts will exceed these, and be less than those – he knows about what they will be. And, with his most delicate instruments and most elaborate calculations, all that the man of science can do, is to reduce the difference between the foreseen and the actual results to an unimportant quantity.

Moreover, it must be borne in mind not only that all the sciences are qualitative in their first stages, – not only that some of them, as Chemistry, have but recently reached the quantitative stage – but that the most advanced sciences have attained to their present power of determining quantities not present to the senses, or not directly measurable, by a slow process of improvement extending through thousands of years. So that science and the knowledge of the uncultured are alike in the nature of their previsions, widely as they differ in range; they possess a common imperfection, though this is immensely greater in the last than in the first; and the transition from the one to the other has been through a series of steps by which the imperfection has been rendered continually less, and the range continually wider.

These facts, that science and the positive knowledge of the uncultured cannot be separated in nature, and that the one is but a perfected and extended form of the other, must necessarily underlie the whole theory of science, its progress, and the relations of its parts to each other. There must be serious incompleteness in any history of the sciences, which, leaving out of view the first steps of their genesis, commences with them only when they assume definite forms. There must be grave defects, if not a general untruth, in a philosophy of the sciences considered in their interdependence and development, which neglects the inquiry how they came to be distinct sciences, and how they were severally evolved out of the chaos of primitive ideas.

Not only a direct consideration of the matter, but all analogy, goes to show that in the earlier and simpler stages must be sought the key to all subsequent intricacies. The time was when the anatomy and physiology of the human being were studied by themselves – when the adult man was analysed and the relations of parts and of functions investigated, without reference either to the relations exhibited in the embryo or to the homologous relations existing in other creatures. Now, however, it has become manifest that no true conceptions, no true generalisations, are possible under such conditions. Anatomists and physiologists now find that the real natures of organs and tissues can be ascertained only by tracing their early evolution; and that the affinities between existing genera can be satisfactorily made out only by examining the fossil genera to which they are allied. Well, is it not clear that the like must be true concerning all things that undergo development? Is not science a growth? Has not science, too, its embryology? And must not the neglect of its embryology lead to a misunderstanding of the principles of its evolution and of its existing organisation?

There are à priori reasons, therefore, for doubting the truth of all philosophies of the sciences which tacitly proceed upon the common notion that scientific knowledge and ordinary knowledge are separate; instead of commencing, as they should, by affiliating the one upon the other, and showing how it gradually came to be distinguishable from the other. We may expect to find their generalisations essentially artificial; and we shall not be deceived. Some illustrations of this may here be fitly introduced, by way of preliminary to a brief sketch of the genesis of science from the point of view indicated. And we cannot more readily find such illustrations than by glancing at a few of the various classifications of the sciences that have from time to time been proposed. To consider all of them would take too much space: we must content ourselves with some of the latest.

Commencing with those which may be soonest disposed of, let us notice first the arrangement propounded by Oken. An abstract of it runs thus: —

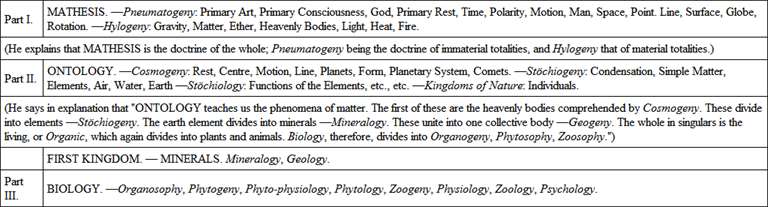
A glance over this confused scheme shows that it is an attempt to classify knowledge, not after the order in which it has been, or may be, built up in the human consciousness; but after an assumed order of creation. It is a pseudo-scientific cosmogony, akin to those which men have enunciated from the earliest times downwards; and only a little more respectable. As such it will not be thought worthy of much consideration by those who, like ourselves, hold that experience is the sole origin of knowledge. Otherwise, it might have been needful to dwell on the incongruities of the arrangements – to ask how motion can be treated of before space? how there can be rotation without matter to rotate? how polarity can be dealt with without involving points and lines? But it will serve our present purpose just to point out a few of the extreme absurdities resulting from the doctrine which Oken seems to hold in common with Hegel, that "to philosophise on Nature is to re-think the great thought of Creation." Here is a sample: —

"Mathematics is the universal science; so also is Physio-philosophy, although it is only a part, or rather but a condition of the universe; both are one, or mutually congruent.

"Mathematics is, however, a science of mere forms without substance. Physio-philosophy is, therefore, mathematics endowed with substance."

From the English point of view it is sufficiently amusing to find such a dogma not only gravely stated, but stated as an unquestionable truth. Here we see the experiences of quantitative relations which men have gathered from surrounding bodies and generalised (experiences which had been scarcely at all generalised at the beginning of the historic period) – we find these generalised experiences, these intellectual abstractions, elevated into concrete actualities, projected back into Nature, and considered as the internal framework of things – the skeleton by which matter is sustained. But this new form of the old realism is by no means the most startling of the physio-philosophic principles. We presently read that,

"The highest mathematical idea, or the fundamental principle of all mathematics is the zero = 0."…

"Zero is in itself nothing. Mathematics is based upon nothing, and, consequently, arises out of nothing.

"Out of nothing, therefore, it is possible for something to arise; for mathematics, consisting of propositions, is something, in relation to 0."

By such "consequentlys" and "therefores" it is, that men philosophise when they "re-think the great thought of Creation." By dogmas that pretend to be reasons, nothing is made to generate mathematics; and by clothing mathematics with matter, we have the universe! If now we deny, as we do deny, that the highest mathematical idea is the zero; – if, on the other hand, we assert, as we do assert, that the fundamental idea underlying all mathematics, is that of equality; the whole of Oken's cosmogony disappears. And here, indeed, we may see illustrated, the distinctive peculiarity of the German method of procedure in these matters – the bastard à priori method, as it may be termed. The legitimate à priori method sets out with propositions of which the negation is inconceivable; the à priori method as illegitimately applied, sets out either with propositions of which the negation is not inconceivable, or with propositions like Oken's, of which the affirmation is inconceivable.

It is needless to proceed further with the analysis; else might we detail the steps by which Oken arrives at the conclusions that "the planets are coagulated colours, for they are coagulated light; that the sphere is the expanded nothing;" that gravity is "a weighty nothing, a heavy essence, striving towards a centre;" that "the earth is the identical, water the indifferent, air the different; or the first the centre, the second the radius, the last the periphery of the general globe or of fire." To comment on them would be nearly as absurd as are the propositions themselves. Let us pass on to another of the German systems of knowledge – that of Hegel.

The simple fact that Hegel puts Jacob Bœhme on a par with Bacon, suffices alone to show that his standpoint is far remote from the one usually regarded as scientific: so far remote, indeed, that it is not easy to find any common basis on which to found a criticism. Those who hold that the mind is moulded into conformity with surrounding things by the agency of surrounding things, are necessarily at a loss how to deal with those, who, like Schelling and Hegel, assert that surrounding things are solidified mind – that Nature is "petrified intelligence." However, let us briefly glance at Hegel's classification. He divides philosophy into three parts: —

1. Logic, or the science of the idea in itself, the pure idea.

2. The Philosophy of Nature, or the science of the idea considered under its other form – of the idea as Nature.

3. The Philosophy of the Mind, or the science of the idea in its return to itself.

Of these, the second is divided into the natural sciences, commonly so called; so that in its more detailed form the series runs thus: – Logic, Mechanics, Physics, Organic Physics, Psychology.

Now, if we believe with Hegel, first, that thought is the true essence of man; second, that thought is the essence of the world; and that, therefore, there is nothing but thought; his classification, beginning with the science of pure thought, may be acceptable. But otherwise, it is an obvious objection to his arrangement, that thought implies things thought of – that there can be no logical forms without the substance of experience – that the science of ideas and the science of things must have a simultaneous origin. Hegel, however, anticipates this objection, and, in his obstinate idealism, replies, that the contrary is true; that all contained in the forms, to become something, requires to be thought: and that logical forms are the foundations of all things.

It is not surprising that, starting from such premises, and reasoning after this fashion, Hegel finds his way to strange conclusions. Out of space and time he proceeds to build up motion, matter, repulsion, attraction, weight, and inertia. He then goes on to logically evolve the solar system. In doing this he widely diverges from the Newtonian theory; reaches by syllogism the conviction that the planets are the most perfect celestial bodies; and, not being able to bring the stars within his theory, says that they are mere formal existences and not living matter, and that as compared with the solar system they are as little admirable as a cutaneous eruption or a swarm of flies.18

Results so outrageous might be left as self-disproved, were it not that speculators of this class are not alarmed by any amount of incongruity with established beliefs. The only efficient mode of treating systems like this of Hegel, is to show that they are self-destructive – that by their first steps they ignore that authority on which all their subsequent steps depend. If Hegel professes, as he manifestly does, to develop his scheme by reasoning – if he presents successive inferences as necessarily following from certain premises; he implies the postulate that a belief which necessarily follows after certain antecedents is a true belief: and, did an opponent reply to one of his inferences, that, though it was impossible to think the opposite, yet the opposite was true, he would consider the reply irrational. The procedure, however, which he would thus condemn as destructive of all thinking whatever, is just the procedure exhibited in the enunciation of his own first principles.

Mankind find themselves unable to conceive that there can be thought without things thought of. Hegel, however, asserts that there can be thought without things thought of. That ultimate test of a true proposition – the inability of the human mind to conceive the negation of it – which in all other cases he considers valid, he considers invalid where it suits his convenience to do so; and yet at the same time denies the right of an opponent to follow his example. If it is competent for him to posit dogmas, which are the direct negations of what human consciousness recognises; then is it also competent for his antagonists to stop him at every step in his argument by saying, that though the particular inference he is drawing seems to his mind, and to all minds, necessarily to follow from the premises, yet it is not true, but the contrary inference is true. Or, to state the dilemma in another form: – If he sets out with inconceivable propositions, then may he with equal propriety make all his succeeding propositions inconceivable ones – may at every step throughout his reasoning draw exactly the opposite conclusion to that which seems involved.

Hegel's mode of procedure being thus essentially suicidal, the Hegelian classification which depends upon it falls to the ground. Let us consider next that of M. Comte.

As all his readers must admit, M. Comte presents us with a scheme of the sciences which, unlike the foregoing ones, demands respectful consideration. Widely as we differ from him, we cheerfully bear witness to the largeness of his views, the clearness of his reasoning, and the value of his speculations as contributing to intellectual progress. Did we believe a serial arrangement of the sciences to be possible, that of M. Comte would certainly be the one we should adopt. His fundamental propositions are thoroughly intelligible; and if not true, have a great semblance of truth. His successive steps are logically co-ordinated; and he supports his conclusions by a considerable amount of evidence – evidence which, so long as it is not critically examined, or not met by counter evidence, seems to substantiate his positions. But it only needs to assume that antagonistic attitude which ought to be assumed towards new doctrines, in the belief that, if true, they will prosper by conquering objectors – it needs but to test his leading doctrines either by other facts than those he cites, or by his own facts differently applied, to at once show that they will not stand. We will proceed thus to deal with the general principle on which he bases his hierarchy of the sciences.

In the second chapter of his Cours de Philosophic Positive, M. Comte says: – "Our problem is, then, to find the one rational order, amongst a host of possible systems." … "This order is determined by the degree of simplicity, or, what comes to the same thing, of generality of their phenomena." And the arrangement he deduces runs thus: Mathematics, Astronomy, Physics, Chemistry, Physiology, Social Physics. This he asserts to be "the true filiation of the sciences." He asserts further, that the principle of progression from a greater to a less degree of generality, "which gives this order to the whole body of science, arranges the parts of each science." And, finally, he asserts that the gradations thus established à priori among the sciences, and the parts of each science, "is in essential conformity with the order which has spontaneously taken place among the branches of natural philosophy;" or, in other words – corresponds with the order of historic development.

Let us compare these assertions with the facts. That there may be perfect fairness, let us make no choice, but take as the field for our comparison, the succeeding section treating of the first science – Mathematics; and let us use none but M. Comte's own facts, and his own admissions. Confining ourselves to this one science, of course our comparisons must be between its several parts. M. Comte says, that the parts of each science must be arranged in the order of their decreasing generality; and that this order of decreasing generality agrees with the order of historical development. Our inquiry must be, then, whether the history of mathematics confirms this statement.

Carrying out his principle, M. Comte divides Mathematics into "Abstract Mathematics, or the Calculus (taking the word in its most extended sense) and Concrete Mathematics, which is composed of General Geometry and of Rational Mechanics." The subject-matter of the first of these is number; the subject-matter of the second includes space, time, motion, force. The one possesses the highest possible degree of generality; for all things whatever admit of enumeration. The others are less general; seeing that there are endless phenomena that are not cognisable either by general geometry or rational mechanics. In conformity with the alleged law, therefore, the evolution of the calculus must throughout have preceded the evolution of the concrete sub-sciences. Now somewhat awkwardly for him, the first remark M. Comte makes bearing upon this point is, that "from an historical point of view, mathematical analysis appears to have risen out of the contemplation of geometrical and mechanical facts." True, he goes on to say that, "it is not the less independent of these sciences logically speaking;" for that "analytical ideas are, above all others, universal, abstract, and simple; and geometrical conceptions are necessarily founded on them."