Six Lectures on Light. Delivered In The United States In 1872-1873

Fig. 16.

§ 11. Application of the Wave-theory to the Phenomena of Diffraction

Of these and of a multitude of similar effects the Emission Theory is incompetent to offer any satisfactory explanation. Let us see how they are accounted for by the Theory of Undulation.

And here, with the view of reaching absolute clearness, I must make an appeal to that faculty the importance of which I have dwelt upon so earnestly here and elsewhere—the faculty of imagination. Figure yourself upon the sea-shore, with a well-formed wave advancing. Take a line of particles along the front of the wave, all at the same distance below the crest; they are all rising in the same manner and at the same rate. Take a similar line of particles on the back of the wave, they are all falling in the same manner and at the same rate. Take a line of particles along the crest, they are all in the same condition as regards the motion of the wave. The same is true for a line of particles along the furrow of the wave.

The particles referred to in each of these cases respectively, being in the same condition as regards the motion of the wave, are said to be in the same phase of vibration. But if you compare a particle on the front of the wave with one at the back; or, more generally, if you compare together any two particles not occupying the same position in the wave, their conditions of motion not being the same, they are said to be in different phases of vibration. If one of the particles lie upon the crest, and the other on the furrow of the wave, then, as one is about to rise and the other about to fall, they are said to be in opposite phases of vibration.

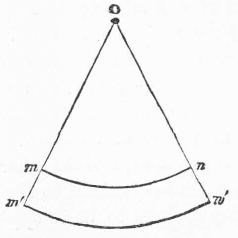
Fig. 17.

There is still another point to be cleared up—and it is one of the utmost importance as regards our present subject. Let O (fig. 17) be a spot in still water which, when disturbed, produces a series of circular waves: the disturbance necessary to produce these waves is simply an oscillation up and down of the water at O. Let m n be the position of the ridge of one of the waves at any moment, and m' n' its position a second or two afterwards. Now every particle of water, as the wave passes it, oscillates, as we have learned, up and down. If, then, this oscillation be a sufficient origin of wave-motion, each distinct particle of the wave m n ought to give birth, to a series of circular waves. This is the important point up to which I wish to lead you. Every particle of the wave m n does act in this way. Taking each particle as a centre, and surrounding it by a circular wave with a radius equal to the distance between m n and m' n', the coalescence of all these little waves would build up the large ridge m' n' exactly as we find it built up in nature. Here, in fact, we resolve the wave-motion into its elements, and having succeeded in doing this we shall have no great difficulty in applying our knowledge to optical phenomena.

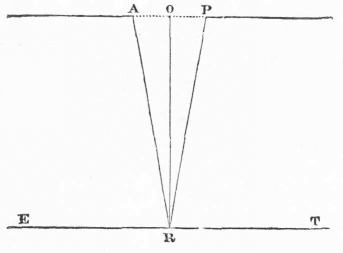
Fig. 18.

Now let us return to our slit, and, for the sake of simplicity, we will first consider the case of monochromatic light. Conceive a series of waves of ether advancing from the first slit towards the second, and finally filling the second slit. When each wave passes through the latter it not only pursues its direct course to the retina, but diverges right and left, tending to throw into motion the entire mass of the ether behind the slit. In fact, as already explained, every point of the wave which fills the slit is itself a centre of a new wave system which is transmitted in all directions through the ether behind the slit. This is the celebrated principle of Huyghens: we have now to examine how these secondary waves act upon each other.

Let us first regard the central band of the series. Let AP (fig. 18) be the width of the aperture held before the eye, grossly exaggerated of course, and let the dots across the aperture represent ether particles, all in the same phase of vibration. Let E T represent a portion of the retina. From O, in the centre of the slit, let a perpendicular O R be imagined drawn upon the retina. The motion communicated to the point R will then be the sum of all the motions emanating in this direction from the ether particles in the slit. Considering the extreme narrowness of the aperture, we may, without sensible error, regard all points of the wave A P as equally distant from R. No one of the partial waves lags sensibly behind the others: hence, at R, and in its immediate neighbourhood, we have no sensible reduction of the light by interference. This undiminished light produces the brilliant central band of the series.

Let us now consider those waves which diverge laterally behind the second slit. In this case the waves from the two sides of the slit have, in order to converge upon the retina, to pass over unequal distances. Let A P (fig. 19) represent, as before, the width of the second slit. We have now to consider the action of the various parts of the wave A P upon a point R' of the retina, not situated in the line joining the two slits.

Fig. 19.

Let us take the particular case in which the difference of path from the two marginal points A, P, to the retina is a whole wave-length of the red light; how must this difference affect the final illumination of the retina?

Let us fix our attention upon the particular oblique line that passes through the centre O of the slit to the retina at R'. The difference of path between the waves which pass along this line and those from the two margins is, in the case here supposed, half a wavelength. Make e R' equal to P R', join P and e, and draw O d parallel to P e. A e is then the length of a wave of light, while A d is half a wave-length. Now the least reflection will make it clear that not only is there discordance between the central and marginal waves, but that every line of waves such as x R', on the one side of O R', finds a line x' R' upon the other side of O R', from which its path differs by half an undulation—with which, therefore, it is in complete discordance. The consequence is, that the light on the one side of the central line will completely abolish the light on the other side of that line, absolute darkness being the result of their coalescence. The first dark interval of our series of bands is thus accounted for. It is produced by an obliquity of direction which causes the paths of the marginal waves to be a whole wave-length different from each other.

When the difference between the paths of the marginal waves is half a wave-length, a partial destruction of the light is effected. The luminous intensity corresponding to this obliquity is a little less than one-half—accurately 0.4—that of the undiffracted light. If the paths of the marginal waves be three semi-undulations different from each other, and if the whole beam be divided into three equal parts, two of these parts will, for the reasons just given, completely neutralize each other, the third only being effective. Corresponding, therefore, to an obliquity which produces a difference of three semi-undulations in the marginal waves, we have a luminous band, but one of considerably less intensity than the undiffracted central band.

With a marginal difference of path of four semi-undulations we have a second extinction of the entire beam, because here the beam can be divided into four equal parts, every two of which quench each other. A second space of absolute darkness will therefore correspond to the obliquity producing this difference. In this way we might proceed further, the general result being that, whenever the direction of wave-motion is such as to produce a marginal difference of path of an even number of semi-undulations, we have complete extinction; while, when the marginal difference is an odd number of semi-undulations, we have only partial extinction, a portion of the beam remaining as a luminous band.

A moment's reflection will make it plain that the wider the slit the less will be the obliquity of direction needed to produce the necessary difference of path. With a wide slit, therefore, the bands, as observed, will be closer together than with a narrow one. It is also plain that the shorter the wave, the less will be the obliquity required to produce the necessary retardation. The maxima and minima of violet light must therefore fall nearer to the centre than the maxima and minima of red light. The maxima and minima of the other colours fall between these extremes. In this simple way the undulatory theory completely accounts for the extraordinary appearance above referred to.

When a slit and telescope are used, instead of the slit and naked eye, the effects are magnified and rendered more brilliant. Looking, moreover, through a properly adjusted telescope with a small circular aperture in front of it, at a distant point of light, the point is seen encircled by a series of coloured bands. If monochromatic light be used, these bands are simply bright and dark, but with white light the circles display iris-colours. If a slit be shortened so as to form a square aperture, we have two series of spectra at right angles to each other. The effects, indeed, are capable of endless variation by varying the size, shape, and number of the apertures through which the point of light is observed. Through two square apertures, with their corners touching each other as at A, Schwerd observed the appearance shown in fig. 20. Adding two others to them, as at B, he observed the appearance represented in fig. 21. The position of every band of light and shade in such figures has been calculated from theory by Fresnel, Fraunhofer, Herschel, Schwerd, and others, and completely verified by experiment. Your eyes could not tell you with greater certainty of the existence of these bands than the theoretic calculation.

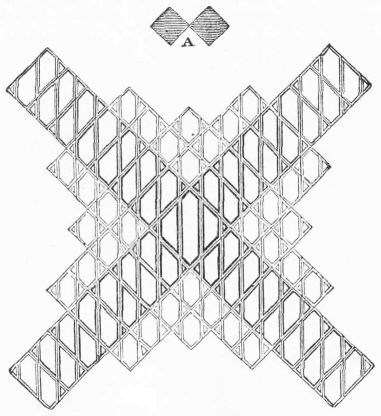
Fig. 20.

The street-lamps at night, looked at through the meshes of a handkerchief, show diffraction phenomena. The diffraction effects obtained in looking through a bird's feathers are, as shown by Schwerd, very brilliant. The iridescence of certain Alpine clouds is also an effect of diffraction which may be imitated by the spores of Lycopodium. When shaken over a glass plate these spores cause a point of light, looked at through the dusted plate, to be surrounded by coloured circles, which rise to actual splendour when the light becomes intense. Shaken in the air the spores produce the same effect. The diffraction phenomena obtained during the artificial precipitation of clouds from the vapours of various liquids in an intensely illuminated tube are, as I have elsewhere shewn, exceedingly fine.

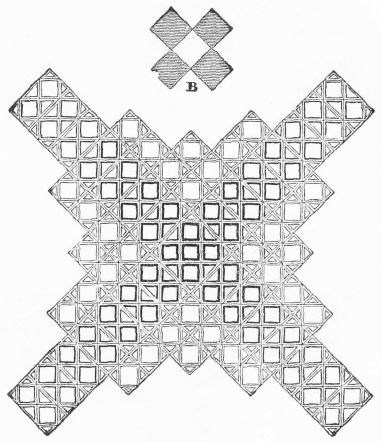
Fig. 21.

One of the most interesting cases of diffraction by small particles that ever came before me was that of an artist whose vision was disturbed by vividly coloured circles. He was in great dread of losing his sight; assigning as a cause of his increased fear that the circles were becoming larger and the colours more vivid. I ascribed the colours to minute particles in the humours of the eye, and ventured to encourage him by the assurance that the increase of size and vividness on the part of the circles indicated that the diffracting particles were becoming smaller, and that they might finally be altogether absorbed. The prediction was verified. It is needless to say one word on the necessity of optical knowledge in the case of the practical oculist.

Without breaking ground on the chromatic phenomena presented by crystals, two other sources of colour may be mentioned here. By interference in the earth's atmosphere, the light of a star, as shown by Arago, is self-extinguished, the twinkling of the star and the changes of colour which it undergoes being due to this cause. Looking at such a star through an opera-glass, and shaking the glass so as to cause the image of the star to pass rapidly over the retina, you produce a row of coloured beads, the spaces between which correspond to the periods of extinction. Fine scratches drawn upon glass or polished metal reflect the waves of light from their sides; and some, being reflected from the opposite sides of the same scratch, interfere with and quench each other. But the obliquity of reflection which extinguishes the shorter waves does not extinguish the longer ones, hence the phenomena of colours. These are called the colours of striated surfaces. They are beautifully illustrated by mother-of-pearl. This shell is composed of exceedingly thin layers, which, when cut across by the polishing of the shell, expose their edges and furnish the necessary small and regular grooves. The most conclusive proof that the colours are due to the mechanical state of the surface is to be found in the fact, established by Brewster, that by stamping the shell carefully upon black sealing-wax, we transfer the grooves, and produce upon the wax the colours of mother-of-pearl.

LECTURE III

RELATION OF THEORIES TO EXPERIENCE

ORIGIN OF THE NOTION OF THE ATTRACTION OF GRAVITATION

NOTION OF POLARITY, HOW GENERATED

ATOMIC POLARITY

STRUCTURAL ARRANGEMENTS DUE TO POLARITY

ARCHITECTURE OF CRYSTALS CONSIDERED AS AN INTRODUCTION

TO THEIR ACTION UPON LIGHT

NOTION OF ATOMIC POLARITY APPLIED TO CRYSTALLINE STRUCTURE

EXPERIMENTAL ILLUSTRATIONS

CRYSTALLIZATION OF WATER

EXPANSION BY HEAT AND BY COLD

DEPORTMENT OF WATER CONSIDERED AND EXPLAINED

BEARINGS OF CRYSTALLIZATION ON OPTICAL PHENOMENA

REFRACTION

DOUBLE REFRACTION

POLARIZATION

ACTION OF TOURMALINE

CHARACTER OF THE BEAMS EMERGENT FROM ICELAND SPAR

POLARIZATION BY ORDINARY REFRACTION AND REFLECTION

DEPOLARIZATION

§ 1. Derivation of Theoretic Conceptions from Experience

One of the objects of our last lecture, and that not the least important, was to illustrate the manner in which scientific theories are formed. They, in the first place, take their rise in the desire of the mind to penetrate to the sources of phenomena. From its infinitesimal beginnings, in ages long past, this desire has grown and strengthened into an imperious demand of man's intellectual nature. It long ago prompted Cæsar to say that he would exchange his victories for a glimpse of the sources of the Nile; it wrought itself into the atomic theories of Lucretius; it impelled Darwin to those daring speculations which of late years have so agitated the public mind. But in no case, while framing theories, does the imagination create its materials. It expands, diminishes, moulds, and refines, as the case may be, materials derived from the world of fact and observation.

This is more evidently the case in a theory like that of light, where the motions of a subsensible medium, the ether, are presented to the mind. But no theory escapes the condition. Newton took care not to encumber the idea of gravitation with unnecessary physical conceptions; but we know that he indulged in them, though he did not connect them with his theory. But even the theory, as it stands, did not enter the mind as a revelation dissevered from the world of experience. The germ of the conception that the sun and planets are held together by a force of attraction is to be found in the fact that a magnet had been previously seen to attract iron. The notion of matter attracting matter came thus from without, not from within. In our present lecture the magnetic force must serve as the portal into a new domain; but in the first place we must master its elementary phenomena.

The general facts of magnetism are most simply illustrated by a magnetized bar of steel, commonly called a bar magnet. Placing such a magnet upright upon a table, and bringing a magnetic needle near its bottom, one end of the needle is observed to retreat from the magnet, while the other as promptly approaches. The needle is held quivering there by some invisible influence exerted upon it. Raising the needle along the magnet, but still avoiding contact, the rapidity of its oscillations decreases, because the force acting upon it becomes weaker. At the centre the oscillations cease. Above the centre, the end of the needle which had been previously drawn towards the magnet retreats, and the opposite end approaches. As we ascend higher, the oscillations become more violent, because the force becomes stronger. At the upper end of the magnet, as at the lower, the force reaches a maximum; but all the lower half of the magnet, from E to S (fig. 22), attracts one end of the needle, while all the upper half, from E to N, attracts the opposite end. This doubleness of the magnetic force is called polarity, and the points near the ends of the magnet in which the forces seem concentrated are called its poles.

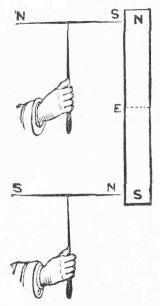
Fig. 22.

What, then, will occur if we break this magnet in two at the centre E? Shall we obtain two magnets, each with a single pole? No; each half is in itself a perfect magnet, possessing two poles. This may be proved by breaking something of less value than the magnet—the steel of a lady's stays, for example, hardened and magnetized. It acts like the magnet. When broken, each half acts like the whole; and when these parts are again broken, we have still the perfect magnet, possessing, as in the first instance, two poles. Push your breaking to its utmost sensible limit—you cannot stop there. The bias derived from observation will infallibly carry you beyond the bourne of the senses, and compel you to regard this thing that we call magnetic polarity as resident in the ultimate particles of the steel. You come to the conclusion that each molecule of the magnet is endowed with this polar force.

Like all other forces, this force of magnetism is amenable to mechanical laws; and, knowing the direction and magnitude of the force, we can predict its action. Placing a small magnetic needle near a bar magnet, it takes a determinate position. That position might be deduced theoretically from the mutual action of the poles. Moving the needle round the magnet, for each point of the surrounding space there is a definite direction of the needle and no other. A needle of iron will answer as well as the magnetic needle; for the needle of iron is magnetized by the magnet, and acts exactly like a steel needle independently magnetized.

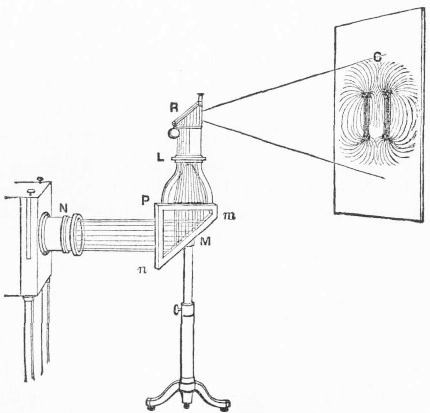
Fig. 23.

N is the nozzle of the lamp; M a plane mirror, reflecting the beam upwards. At P the magnets and iron filings are placed; L is a lens which forms an image of the magnets and filings; and R is a totally reflecting prism, which casts the image G upon the screen.

If we place two or more needles of iron near the magnet, the action becomes more complex, for then the needles are not only acted on by the magnet, but they act upon each other. And if we pass to smaller masses of iron—to iron filings, for example—we find that they act substantially as the needles, arranging themselves in definite forms, in obedience to the magnetic action.

Placing a sheet of paper or glass over a bar magnet and showering iron filings upon the paper, I notice a tendency of the filings to arrange themselves in determinate lines. They cannot freely follow this tendency, for they are hampered by the friction against the paper. They are helped by tapping the paper; each tap releasing them for a moment, and enabling them to follow their tendencies. But this is an experiment which can only be seen by myself. To enable you all to see it, I take a pair of small magnets and by a simple optical arrangement throw the magnified images of the magnets upon the screen. Scattering iron filings over the glass plate to which the small magnets are attached, and tapping the plate, you see the arrangement of the iron filings in those magnetic curves which have been so long familiar to scientific men (fig. 23).

(By a very ingenious device, Professor Mayer, of Hoboken, has succeeded in fixing and photographing the magnetic curves. I am indebted to his kindness for the annexed beautiful illustration, fig. 24.)

The aspect of these curves so fascinated Faraday that the greater portion of his intellectual life was devoted to pondering over them. He invested the space through which they run with a kind of materiality; and the probability is that the progress of science, by connecting the phenomena of magnetism with the luminiferous ether, will prove these 'lines of force,' as Faraday loved to call them, to represent a condition of this mysterious substratum of all radiant action.

It is not, however, the magnetic curves, as such, but their relationship to theoretic conceptions, that we have now to consider. By the action of the bar magnet upon the needle we obtain the notion of a polar force; by the breaking of the strip of magnetized steel we attain the notion that polarity can attach itself to the ultimate particles of matter. The experiment with the iron filings introduces a new idea into the mind; the idea, namely, of structural arrangement. Every pair of filings possesses four poles, two of which are attractive and two repulsive. The attractive poles approach, the repulsive poles retreat; the consequence being a certain definite arrangement of the particles with reference to each other.

§ 2. Theory of Crystallization

Now this idea of structure, as produced by polar force, opens a way for the intellect into an entirely new region, and the reason you are asked to accompany me into this region is, that our next inquiry relates to the action of crystals upon light. Prior to speaking of this action, I wish you to realise intellectually the process of crystalline architecture. Look then into a granite quarry, and spend a few minutes in examining the rock. It is not of perfectly uniform texture. It is rather an agglomeration of pieces, which, on examination, present curiously defined forms. You have there what mineralogists call quartz, you have felspar, you have mica. In a mineralogical cabinet, where these substances are preserved separately, you will obtain some notion of their forms. You will see there, also, specimens of beryl, topaz, emerald, tourmaline, heavy spar, fluor-spar, Iceland spar—possibly a full-formed diamond, as it quitted the hand of Nature, not yet having got into the hands of the lapidary.

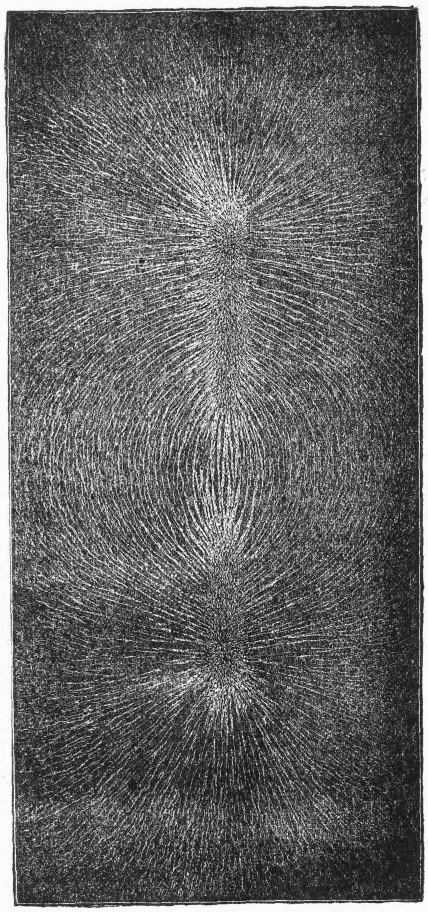
Fig. 24.

These crystals, you will observe, are put together according to law; they are not chance productions; and, if you care to examine them more minutely, you will find their architecture capable of being to some extent revealed. They often split in certain directions before a knife-edge, exposing smooth and shining surfaces, which are called planes of cleavage; and by following these planes you sometimes reach an internal form, disguised beneath the external form of the crystal. Ponder these beautiful edifices of a hidden builder. You cannot help asking yourself how they were built; and familiar as you now are with the notion of a polar force, and the ability of that force to produce structural arrangement, your inevitable answer will be, that those crystals are built by the play of polar forces with which their molecules are endowed. In virtue of these forces, molecule lays itself to molecule in a perfectly definite way, the final visible form of the crystal depending upon this play of its ultimate particles.